All Algebra II Resources
Example Questions
Example Question #1 : Adding And Subtracting Logarithms
Simplify the following logarithmic expression:
Each term can be simplified as follows:
Combining these gives the answer:
Example Question #1 : Adding And Subtracting Logarithms
Simplify the expression using logarithmic identities.
The expression cannot be simplified
The logarithm of a fraction is equal to the logarithm of the numerator minus the logarithm of the denominator.
If we encounter two logarithms with the same base, we can likely combine them. In this case, we can use the reverse of the above identity.
Example Question #1 : Adding And Subtracting Logarithms
Use logarithmic properties to simplify this expression:
Use the sum/product rule to combine the first 2 terms:
Use the difference/quotient rule to combine the remaining terms:
Example Question #2 : Adding And Subtracting Logarithms
Expand the following logarithmic expression into a list of sums or subtractions of logarithms:
One important property of logarithms is that multiplication inside the logarithm is the same thing as addition outside of it. In the same way division is "the same" as subtraction in logarithms. So our expression is the same as
But also, exponents can be moved outside in the same way. 


Example Question #5 : Adding And Subtracting Logarithms
What is the value of 
Remember the rules of logarithms:

This means we can simplify it as follows:
The logarithm of anything with the same base is always 

Example Question #1 : Adding And Subtracting Logarithms
Which of the following is another way to express

Use the rule
therefore
Example Question #1 : Adding And Subtracting Logarithms
Which is another way of expressing

Use the rule:
therefore
Example Question #4 : Adding And Subtracting Logarithms
Simplify
This problem can be solved using the properties of logs. When two logs are being subtracted from each other, it is the same thing as dividing two logs together. Remember that to use this rule, the logs must have the same base in this case 
Example Question #9 : Adding And Subtracting Logarithms
Condense this logarithm:
In order to solve this problem you must understand the product property of logarithms 

first move the constants in front of the logarithmic functions to their proper place using the power rule.
next factor out the logarithmic equation:
change the fractional exponent to a radical
Example Question #1 : Adding And Subtracting Logarithms
Expand
The rule for expanding and dividing logarithms is that you can subtract the terms inside the log. In this case, the question is not asking for an actual number, but just what the expanded version would be. Therefore you separate the terms inside the log by subtracting the denominator from the numerator. Therefore the answer is
Certified Tutor
Certified Tutor
All Algebra II Resources














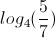




















































![\log_{10}\left[\sqrt{x}+\log_{10}(x+1)^3\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/439782/gif.latex)
![\log_{10}\left[\sqrt{x}(x+1)^3\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/439779/gif.latex)
![\log_{10}\left[\sqrt{x}+3\log_{10}(x+1)\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/439780/gif.latex)

![\frac{1}{2}\log_{10}\left[x+\log_{10}(x+1)^3\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/439781/gif.latex)


![\log_{10}[x^\frac{1}{2}+(x+1)^3]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/434518/gif.latex)
![\log_{10}[\sqrt{x}(x+1)^3]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/434499/gif.latex)









