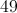All Algebra II Resources
Example Questions
Example Question #13 : Quadratic Equations And Inequalities
Given 
In general, the discriminant is 
In this particual case 
Plug in these three values and simplify:
Example Question #1 : Discriminants
The equation
has two imaginary solutions.
For what positive integer values of 
All positive integers
For the equation
to have two imaginary solutions, its discriminant 


Therefore, if 

Example Question #1 : Discriminants
The equation
has two real solutions.
For what positive integer values of 
All positive integers
For the equation
to have two real solutions, its discriminant 


Therefore, if 
Example Question #4 : Discriminants
What is the discriminant of the following quadratic equation? Are its roots real?
The equation's discriminant is 
The equation's discriminant is 
The equation's discriminant is 
The equation's roots are not real; therefore, it does not have a discriminant.
The equation's discriminant is 
The equation's discriminant is 
The "discriminant" is the name given to the expression that appears under the square root (radical) sign in the quadratic formula, 








Example Question #5 : Discriminants
Find the discriminant, 
Remember the quadratic formula:

The discriminant in the quadratic formula is the term that appears under the square root symbol. It tells us about the nature of the roots.
So, to find the discriminant, all we need to do is compute 

We get 
Example Question #2 : Discriminants
Choose the answer that is the most correct out of the following options.
How many solutions does the function 
2 imaginary solutions
2 real solutions
No solution
1 real solution; 1 imaginary solution
1 real solution
2 real solutions
The number of roots can be found by looking at the discriminant. The discriminant is determined by 




Example Question #2 : Discriminants
Determine the discriminant of the following quadratic equation 
The discriminant is found using the equation 





Example Question #8 : Discriminants
What is the discriminant for the function 
Given that quadratics can be written as 

Example Question #1 : Discriminants
How many solutions does the quadratic 
no solutions






The discrimiant will determine how many solutions a quadratic has. If the discriminant is positive, then there are two real solutions. If it is negative then there are two immaginary solutions. If it is equal to zero then there is one repeated solution.
Given that quadratics can be written as 

The discriminant is positive; therefore, there are two real solutions to this quadratic.
Example Question #10 : Discriminants
How many real roots are there to the following equation:
None of the above
This is using the discriminant to find roots. The discriminant as you recall is
If you get a negative number you have no real roots, if you get zero you have one, and if you get a positive number you have two real roots.
So plug in your numbers:
Because you get a negative number you have zero real roots.
Certified Tutor
All Algebra II Resources