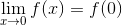We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

AP Calculus AB : Understanding continuity in terms of limits

Study concepts, example questions & explanations for AP Calculus AB

Create An Account

All AP Calculus AB Resources

3 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #2 : Continunity As A Property Of Functions

If $\lim_{x \to 0} f(x)$ exists,

Possible Answers:

f(0) exists and $\lim_{x \to 0}f(x) = f(0)$

f(x) must be continuous at all values.

We cannot conclude any of the other answers.

f(x) must be continuous at x=0 .

$\lim_{x \to \infty} f(x)$ exists.

Correct answer:

We cannot conclude any of the other answers.

Explanation:

Unless we are explicitly told so, via graph, information, or otherwise, we cannot assume f(x) is continuous at x=0 unless $\lim_{x \to 0}f(x) = f(0)$ , which is required for f(x) to be continuous at x=0 .

We cannot assume anything about the existence of $\lim_{x \to \infty} f(x)$ , because we do not know what f(x) is, or its end behavior.

Report an Error

Example Question #4 : Continunity As A Property Of Functions

$f(x) = \left\{\begin{matrix} x^{2}- 3x+ 17,& x<2 \\ 17, & x=2\\ x^{2}- 4x+ 16,&x > 2 \end{matrix}\right.$

Which of the following is equal to $\lim_{x\rightarrow 2 }f(x)$ ?

Possible Answers:

$\lim_{x\rightarrow 2 }f(x)$ does not exist.

13.5

Correct answer:

$\lim_{x\rightarrow 2 }f(x)$ does not exist.

Explanation:

The limit of a function as approaches a value $x_{0}$ exists if and only if the limit from the left is equal to the limit from the right; the actual value of $f(x_{0})$ is irrelevant. Since the function is piecewise-defined, we can determine whether these limits are equal by finding the limits of the individual expressions. These are both polynomials, so the limits can be calculated using straightforward substitution:

$\lim_{x\rightarrow 2^{-} }f(x)= \lim_{x\rightarrow 2^{-} } (x^{2}- 3x+ 17) = 2^{2}- 3(2)+ 17 = 15$

$\lim_{x\rightarrow 2^{+} }f(x) = \lim_{x\rightarrow 2^{+} }(x^{2}- 4x+ 16) = 2^{2}- 4(2)+16 = 12$

$\lim_{x\rightarrow 2 }f(x)$ does not exist, because $\lim_{x\rightarrow 2^{-} }f(x) \ne \lim_{x\rightarrow 2^{+} }f(x)$ .

Report an Error

Example Question #2 : Continunity As A Property Of Functions

Determine any points of discontinuity for the function:

$f(x)=\frac{2}{ln(x^2)}$

Possible Answers:

x=-1,1

x=0

x=-1,0.1

x=0, 1

x=-1

Correct answer:

x=-1,1

Explanation:

For a function to be continuous the following criteria must be met:

The function must exist at the point (no division by zero, asymptotic behavior, negative logs, or negative radicals).
The limit must exist.
The point must equal the limit. (Symbolically, $\lim_{x\rightarrow c}f(x)=f(c)$ ).

It is easiest to first find any points where the function is undefined. Since our function involves a fraction and a natural log, we must find all points in the domain such that the natural log is less than or equal to zero, or points where the denominator is equal to zero.

To find the values that cause the natural log to be negative we set

ln(x^2)=0

$e^{ln(x^2)}=e^{0}$

x^2=1

$x=\pm1$

Therefore, those x values will yield our points of discontinuity. Normally, we would find values where the natural log is negative; however, for all x^2 the function is positive.

Report an Error

View AP Calculus AB Tutors

Muhammad
Certified Tutor

The University of Texas at Dallas, Bachelor of Science, Mechanical Engineering.

View AP Calculus AB Tutors

Pavan
Certified Tutor

University of Tennessee, Knoxville, Master's/Graduate, Mechanical Engineering. Pennsylvania State University, Master's/Gradua...

View AP Calculus AB Tutors

Omar
Certified Tutor

Arizona State University, Bachelors, Civil Engineering.

All AP Calculus AB Resources

3 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Spanish Tutors in Phoenix, Statistics Tutors in Houston, Reading Tutors in Chicago, ISEE Tutors in Washington DC, Physics Tutors in Seattle, Biology Tutors in Seattle, Chemistry Tutors in Houston, French Tutors in Los Angeles, SAT Tutors in Atlanta, Reading Tutors in Los Angeles

Popular Courses & Classes

GRE Courses & Classes in New York City, Spanish Courses & Classes in San Francisco-Bay Area, SSAT Courses & Classes in San Francisco-Bay Area, LSAT Courses & Classes in Philadelphia, GMAT Courses & Classes in Houston, ACT Courses & Classes in New York City, SAT Courses & Classes in Seattle, ACT Courses & Classes in Boston, Spanish Courses & Classes in Philadelphia, SAT Courses & Classes in Miami

Popular Test Prep

ACT Test Prep in Los Angeles, SSAT Test Prep in San Francisco-Bay Area, GRE Test Prep in New York City, SSAT Test Prep in Boston, ACT Test Prep in Dallas Fort Worth, MCAT Test Prep in Denver, GMAT Test Prep in Houston, GMAT Test Prep in New York City, MCAT Test Prep in Washington DC, GMAT Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

AP Calculus AB : Understanding continuity in terms of limits

Study concepts, example questions & explanations for AP Calculus AB

All AP Calculus AB Resources

Example Questions

Example Question #2 : Continunity As A Property Of Functions

Example Question #4 : Continunity As A Property Of Functions

Example Question #2 : Continunity As A Property Of Functions

All AP Calculus AB Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: