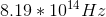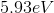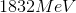All AP Physics 2 Resources
Example Questions
Example Question #1 : Electromagnetic Spectrum And Radiation
Determine the frequency of orange light, 
None of these
Using
Converting 

Example Question #1 : Principles Of Quantum Mechanics
Determine the photon energy of orange light, 
None of these
Using




Converting 

Example Question #2 : Electromagnetic Spectrum And Radiation
A beam of light is traveling at approximately 


The wavelength of the light beam doesn't depend on the speed through the medium. As such, as can solve for wavelength 
Where 

Example Question #1 : Quantum And Nuclear Physics
A physicist is trying to determine the identity of a metal by experimentally finding its work function and then comparing the experimental value to a list of known values. If the physicist shines light with a frequency of 

To determine the work function of the metal, we can begin by calculating the kinetic energy of the electron from its speed.
Then, convert joules into electron volts:
Next, we'll calculate the energy of the light by using its frequency according to the equation:
Then, we can convert this energy into electron volts:
And finally, we can use the equation for work function:
Example Question #1 : Photons And Photon Energy
An atomic transition takes place from 

The energy difference between the second excited state 

Since the energy difference is in electron volts, they need to be converted to Joules. Another convenient formula is to notice that since 

Example Question #6 : Principles Of Quantum Mechanics
It is possible that electrons can be ejected from a metal plate by light. Which of the following properties of light accounts for this phenomena?
Intensity
Frequency
Amplitude
Speed
Electric force
Frequency
The ejection of electrons from a metal is known as the photoelectric effect. In fact, it was a key discovery that led to the acceptance of the particle nature of light, because the wave nature of light could not account for this finding.
The key that allowed scientists to find this out was that the speed of electrons ejected from the metal was dependent on the color of the light. The intensity of light had no effect on the speed at which electrons were ejected.
As a part of developing this theory, scientists referred to individual discrete particles of light as photons. In order to eject electrons from the metal, individual photons needed to have a sufficient amount of energy. The intensity of light (with a greater amplitude of its waves) represents a case in which photons are striking the metal at a greater rate. But, the individual energy of the photons could be too low in such a situation, hence why intensity is not the key property here. Instead, a higher frequency of light allows each individual photon to have greater energy - enough to knock the metal's electrons off. Furthermore, as the frequency of the light increases, the energy of the individual photons increases and the kinetic energy of the ejected electrons increases as well. The expression for the energy of a photon is shown as follows.
Example Question #7 : Principles Of Quantum Mechanics
How much energy is contained in a particle that has a mass of 
This is an example of one of Einstein's greatest ideas: the relation between the mass of an object/particle, and the energy contained by the mass. This is given as
In order to calculate the energy in our particle, we must make sure that the mass is in units of 
Now we can plug in numbers to our equation and solve for energy.
Example Question #1 : Principles Of Quantum Mechanics
Suppose that the mass of a neutral Uranium atom is measured and found to be 

In this question, we're presented with information concerning the mass of a uranium atom. We're told two values: the mass of a uranium atom as measured, and the predicted mass of a uranium atom. We're then asked to determine the nuclear binding energy for uranium.
In order to solve this question, we have to realize the significance of the discrepancy between the observed and predicted mass of uranium. The predicted mass is calculated by adding up the individual masses of each constituent proton, electron, and neutron. However, the reason why the measured mass is less than the predicted mass is due to energy-mass equivalence. When the constituent protons and neutrons come together to form the nucleus, some of their mass is converted into energy, and it is this energy which holds these constituent nucleons together. Because some of the mass is converted into energy, the observed mass is less than what we would predict.
Now that we understand why there is a discrepancy between observed and predicted mass, we can calculate the nuclear binding energy by using Einstein's famous equation.
This equation states that the nuclear binding energy is equal to the difference between observed and predicted mass, multiplied by the speed of light squared. So to solve for energy, we can plug in the values given to us.
Example Question #9 : Principles Of Quantum Mechanics
Two grams of helium are completely converted into energy and used to power a 
The energy from the two grams of helium can be found using
This energy can then be equated to the man's kinetic energy, which can then be used to find the man's velocity.
Example Question #1 : Principles Of Quantum Mechanics
If the combination of protons and neutrons in an atom's nucleus results in a mass defect of 
In this question, we're given the mass defect of an atom's nucleus and are asked to find the binding energy for this atom.
To begin with, it's important to understand that when protons and neutrons come to be held together within the nucleus of an atom, there is a tremendously powerful force holding them together. This incredibly large force accounts for the mass defect. In other words, the total mass of the nucleus is smaller than the sum of the individual masses of the protons and neutrons that make up that nucleus, and this is due to the strong force.
Einstein's mass-energy equivalence explains the observable mass defect; the mass lost is converted into an enormous amount of energy according to the following equation.
But first, we'll need to convert the mass given to us in the question stem into grams.
Furthermore, because we know the value for the speed of light, we can use this information to solve for the binding energy.
Certified Tutor
All AP Physics 2 Resources