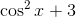We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

High School Math : Trigonometric Identities

Study concepts, example questions & explanations for High School Math

Create An Account

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Using Basic And Definitional Identities

Trig_id

What is the $\tan$ of $\theta$ ?

Possible Answers:

$\frac{a}{h}$

$\frac{a}{o}$

$\frac{o}{h}$

$\frac{o}{a}$

Correct answer:

$\frac{o}{a}$

Explanation:

When working with basic trigonometric identities, it's easiest to remember the mnemonic: SOHCAHTOA .

$\sin=\frac{opposite}{hypotenuse}$

$\cos=\frac{adjacent}{hypotenuse}$

$\tan=\frac{opposite}{adjacent}$

When one names the right triangle, the opposite side is opposite to the angle, the adjacent side is next to the angle, and the hypotenuse spans the two legs of the right angle.

Report an Error

Example Question #2 : Trigonometric Identities

Simplify $(1-\cos^2\theta )\cdot\cot\theta\cdot\csc^2\theta$ .

Possible Answers:

$\cos^2\theta$

$\sin\theta$

$\sin^2\theta$

$\cot\theta$

$\tan\theta$

Correct answer:

$\cot\theta$

Explanation:

Simplifying trionometric expressions or identities often involves a little trial and error, so it's hard to come up with a strategy that works every time. A lot of times you have to try multiple strategies and see which one helps.

Often, if you have any form of $\tan\theta,$ $\cot\theta,$ $\csc\theta,$ or $\sec\theta,$ in an expression, it helps to rewrite it in terms of sine and cosine. In this problem, we can use the identities $\cot\theta=\frac{\cos\theta }{\sin\theta }$ and $\csc\theta=\frac{1}{\sin\theta }$ .

$(1-\cos^2\theta )\cdot\cot\theta\cdot\csc^2\theta=(1-\cos^2\theta )\cdot\frac{\cos\theta }{\sin\theta }\cdot(\frac{1}{\sin\theta})^2$

$=(1-\cos^2\theta )\cdot\frac{\cos\theta }{\sin\theta }\cdot\frac{1}{\sin^2\theta}$ .

This doesn't seem to help a whole lot. However, we should recognize that $(1-\cos^2\theta )=\sin^2\theta$ because of the Pythagorean identity $\sin^2\theta+\cos^2\theta=1$ .

$(1-\cos^2\theta )\cdot\frac{\cos\theta }{\sin\theta }\cdot\frac{1}{\sin^2\theta}=(\sin^2\theta )\cdot\frac{\cos\theta }{\sin\theta }\cdot\frac{1}{\sin^2\theta}$

We can cancel the $\sin^2\theta$ terms in the numerator and denominator.

$(\sin^2\theta )\cdot\frac{\cos\theta }{\sin\theta }\cdot\frac{1}{\sin^2\theta}=\frac{\cos\theta }{\sin\theta }=\cot\theta$ .

Report an Error

Example Question #1 : Using Basic And Definitional Identities

Trig_id

What is the $\sin$ of $\theta$ ?

Possible Answers:

$\frac{h}{o}$

$\frac{o}{h}$

$\frac{h}{a}$

$\frac{o}{a}$

$\frac{a}{h}$

Correct answer:

$\frac{o}{h}$

Explanation:

When working with basic trigonometric identities, it's easiest to remember the mnemonic: SOHCAHTOA .

$\sin=\frac{opposite}{hypotenuse}$

$\cos=\frac{adjacent}{hypotenuse}$ ,

$\tan=\frac{opposite}{adjacent}$

When one names the right triangle, the opposite side is opposite to the angle, the adjacent side is next to the angle, and the hypotenuse spans the two legs of the right angle.

Report an Error

Example Question #2 : Using Basic And Definitional Identities

Trig_id

What is the $\cos$ of $\theta$ ?

Possible Answers:

$\frac{a}{h}$

$\frac{o}{a}$

$\frac{o}{h}$

$\frac{h}{o}$

$\frac{h}{a}$

Correct answer:

$\frac{a}{h}$

Explanation:

When working with basic trigonometric identities, it's easiest to remember the mnemonic: SOHCAHTOA .

$\sin=\frac{opposite}{hypotenuse}$

$\cos=\frac{adjacent}{hypotenuse}$ ,

$\tan=\frac{opposite}{adjacent}$

When one names the right triangle, the opposite side is opposite to the angle, the adjacent side is next to the angle, and the hypotenuse spans the two legs of the right angle.

Report an Error

Example Question #1 : Trigonometric Identities

Simplify

$\cot(x)\times{\sin(x) }$

Possible Answers:

$\arcsin(x)$

$\tan(x)$

$\csc(x)$

$\cos(x)$

$\arcsin(\pi )$

Correct answer:

$\cos(x)$

Explanation:

$\cot(x) = \cos(x)/\sin(x)$ . Thus: $[\cos(x)/\sin(x)]\times\sin(x)=\cos(x)$

Report an Error

Example Question #2 : Trigonometric Identities

Simplify

$\frac{cot (x)\cdot tan(x)}{cos(x)}$

Possible Answers:

$\cos(x)$

$\cot(x)/sec(x)$

$\sec(x)$

$\cos(x)\times\tan(x)$

$\csc(x)$

Correct answer:

$\sec(x)$

Explanation:

$\cot(x)\times\tan(x) = 1$

and

$1/(\cos(x)) = \sec(x)$ .

Report an Error

Example Question #6 : Trigonometric Identities

Simplify $\frac{\cos^2x-1}{\sin x}$ .

Possible Answers:

$-\sin x$

$\sin^2 x$

$\sin x$

$\sin x\cos x$

$\tan x$

Correct answer:

$-\sin x$

Explanation:

Remember that $\sin^2x+\cos^2 x=1$ . We can rearrange this to simplify our given equation:

$\frac{\cos^2x-1}{\sin x}$

$=\frac{-\sin^2x}{\sin x}=-\sin x$

Report an Error

Example Question #3 : Trigonometric Identities

Simplify:

$\sin^2x+\cos^2x+\tan^2x$

Possible Answers:

This is the most simplified version.

$\sin x\cos x$

$2\cos x+\cot x$

$\sec^2x$

$\csc^2 x$

Correct answer:

$\sec^2x$

Explanation:

Whenever you see a trigonometric function squared, start looking for a Pythagorean identity.

The two identities used in this problem are $\sin^2 x + \cos ^2 x=1$ and $\tan^2 x +1 =\sec^2 x$ .

Substitute and solve.

$\sin^2x+\cos^2x+\tan^2x=?$

$(\sin^2x+\cos^2x)+\tan^2x=?$

$(1)+\tan^2x=\sec^2 x$

Report an Error

Example Question #1 : Using Identities Of Squared Functions

Factor and simplify 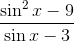$\frac{\sin^2x-9}{\sin x-3}$ .

Possible Answers:

This is already it's most reduced form.

$\sin x+3$

$\cos^2 x+3$

$\sin x \cos x$

$\csc x$

Correct answer:

$\sin x+3$

Explanation:

To reduce 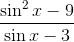$\frac{\sin^2x-9}{\sin x-3}$ , factor the numerator: $\frac{(\sin x-3)(\sin x+3)}{\sin x-3}$

Notice that we can cancel out a $\sin x -3$ .

This leaves us with 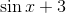$\sin x +3$ .

Report an Error

Example Question #3 : Trigonometric Identities

Simplify $\sin x\cot x$ .

Possible Answers:

$\cos^2x$

$cos \, x$

$\sin x \cos x$

$\tan x$

$\csc x$

Correct answer:

$cos \, x$

Explanation:

To simplify $\sin x\cot x$ , break them into their SOHCAHTOA parts:

$\frac{\text{opposite}}{\text{hypotenuse}}*\frac{\text{adjacent}}{\text{opposite}}$ .

Notice that the opposite's cancel out, leaving $\frac{\text{adjacent}}{\text{hypotenuse}}=\cos x$ .

Report an Error

View Tutors

Fabrianne
Certified Tutor

University of Washington-Seattle Campus, Bachelors, Electrical Engineering.

View Tutors

Ramis
Certified Tutor

Broward College, Associate in Arts, History. University of Central Florida, Bachelor in Arts, History.

View Tutors

Toniann
Certified Tutor

Florida International University, Masters in Education, Curriculum and Instruction.

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

GMAT Tutors in San Diego, Chemistry Tutors in Chicago, ACT Tutors in Los Angeles, SAT Tutors in Atlanta, Chemistry Tutors in Washington DC, ISEE Tutors in Chicago, GMAT Tutors in Washington DC, Biology Tutors in San Francisco-Bay Area, MCAT Tutors in San Diego, Algebra Tutors in San Francisco-Bay Area

Popular Courses & Classes

ACT Courses & Classes in Dallas Fort Worth, MCAT Courses & Classes in Dallas Fort Worth, GMAT Courses & Classes in Boston, MCAT Courses & Classes in San Diego, GRE Courses & Classes in Seattle, GMAT Courses & Classes in San Francisco-Bay Area, ACT Courses & Classes in Washington DC, LSAT Courses & Classes in Boston, SAT Courses & Classes in Los Angeles, LSAT Courses & Classes in Atlanta

Popular Test Prep

GMAT Test Prep in San Francisco-Bay Area, GRE Test Prep in Denver, SSAT Test Prep in San Diego, GMAT Test Prep in Boston, GRE Test Prep in Washington DC, SSAT Test Prep in Miami, MCAT Test Prep in San Francisco-Bay Area, SSAT Test Prep in Boston, ISEE Test Prep in Boston, ACT Test Prep in Dallas Fort Worth

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

High School Math : Trigonometric Identities

Study concepts, example questions & explanations for High School Math

All High School Math Resources

Example Questions

Example Question #1 : Using Basic And Definitional Identities

Example Question #2 : Trigonometric Identities

Example Question #1 : Using Basic And Definitional Identities

Example Question #2 : Using Basic And Definitional Identities

Example Question #1 : Trigonometric Identities

Example Question #2 : Trigonometric Identities

Example Question #6 : Trigonometric Identities

Example Question #3 : Trigonometric Identities

Example Question #1 : Using Identities Of Squared Functions

Example Question #3 : Trigonometric Identities

All High School Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: