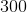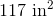All MAP 6th Grade Math Resources
Example Questions
Example Question #1 : Operations And Algebraic Thinking
Solve for 
In order to solve this equation we need to isolate the variable to one side. Do not forget to perform the same operation on both sides of the equation.
Divide each side of the equation by 
Solve.
Example Question #2 : Operations And Algebraic Thinking
Read the following scenario:
A barista has to make sixty pounds of a special blend of coffee at Moonbucks, using Hazelnut Happiness beans and Pecan Delight beans. If there are fourteen fewer pounds of Hazelnut Happiness beans in the mixture than Pecan Delight beans, then how many pounds of each will she use?
If 
Since there are fourteen fewer pounds of Hazelnut Happiness beans in the mixture than Pecan Delight beans , then the number of pounds of Hazelnut Happiness beans is fourteen subtracted from 

Add the number of pounds of Pecan Delight beans, 


This translates to the following equation:
Example Question #3 : Operations And Algebraic Thinking
What is 
When expanding an exponent, we multiply the base by itself for the number indicated by the exponential value.
In this case, our base number is 



Remember, the question asks us for the multiplication problem, not the answer to 

Example Question #1 : Map 6th Grade Math
Candidate A receives 


In order to solve this problem we need to create a ratio with the given information. It says that for every 

Now substitute in the given numbers.
We know that candidate B received 
Now, use the original relationship to create a proportion and solve for the number of votes that candidate A received.
Cross multiply and solve for 
Simplify and solve.
Example Question #2 : Map 6th Grade Math
Use the distributive property to express the sum 
The distributive property can be used to rewrite an expression. When we use this property we will identify and pull out the greatest common factor of each of the addends. Then we can create a quantity that represents the sum of two whole numbers with no common factor multiplied by their greatest common factor.
In this case, the greatest common factor shared by each number is:
After we reduce each addend by the greatest common factor we can rewrite the expression:
Example Question #3 : Map 6th Grade Math
Which value for 
All of the choices are correct
All of the choices are correct
We can use substitution to determine which value of 
Because we are looking for a value of 

Example Question #4 : Map 6th Grade Math
What is the area of the right triangle in the following figure?

There are several different ways to solve for the area of a right triangle. In this lesson, we will transform the right triangle into a rectangle, use the the simpler formula for area of a rectangle to solve for the new figure's area, and divide this area in half in order to solve for the area of the original figure.
First, let's transform the triangle into a rectangle:

Second, let's remember that the formula for area of a rectangle is as follows:
Substitute in our side lengths.
Last, notice that our triangle is exactly half the size of the rectangle that we made. This means that in order to solve for the area of the triangle we will need to take half of the area of the rectangle, or divide it by 
Thus, the area formula for a right triangle is as follows:

Example Question #5 : Map 6th Grade Math
What is the volume of the rectangular prism in the following figure?

The formula used to find volume of a rectangular prism is as follows:
Substitute our side lengths:
Remember, volume is always written with cubic units because volume is how many cubic units can fit inside of a figure.
Example Question #6 : Map 6th Grade Math
Hydraulic fracturing is a process used by gas companies to rupture and collects pockets of gas trapped within pockets of shale rock. A particular shale fracking site is 

In order to solve this question, we need to first recall how to find the area of a rectangle.
Substitute in the given values in the equation and solve for 
Divide both sides by
Dividing by a fraction is the same as multiplying by its inverse or reciprocal.
Find the reciprocal of
Simplify and rewrite.
Multiply and solve.
Reduce.
The width of the fracking site is
Example Question #7 : Map 6th Grade Math
Find the range of this set of numbers:
First order the numbers from least to greatest:
Then subtract the smallest number from the largest number:
Answer:
All MAP 6th Grade Math Resources