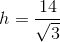All ACT Math Resources
Example Questions
Example Question #1 : How To Find The Perimeter Of A Hexagon

The figure above is a regular hexagon. 
What is the perimeter of the figure above?
You can redraw the figure given to notice the little equilateral triangle that is formed within the hexagon. Since a hexagon can have the 



Now, the 




We know, then, that:
Or,
Now, this is only half of the size of the hexagon's side. Therefore, the full side length is 
Since this is a regular hexagon, all of the sides are of equal length. This means that your total perimeter is 

Example Question #31 : Geometry
What is the perimeter of a regular hexagon with an area of 
The area of a regular hexagon is defined by the equation:


This is derived from the fact that the regular hexagon can be split up into 
To visualize this, consider the drawing:

Each triangle formed like this will be equilateral. It is easiest to remember this relationship and memorize the general area equation for equilateral triangles. (It is useful in many venues!)
So, for your data, you know:
Solving for 
This means that
Therefore, the perimeter of the figure is equal to 

Example Question #1 : How To Find The Perimeter Of A Hexagon
Find the perimeter of a hexagon with a side length of 
There are 6 sides in a hexagon.
Therefore, given a side length of 16, the perimeter is:
Example Question #2 : How To Find The Perimeter Of A Hexagon
The side length of a hexagon is 
Write the formula for the perimeter of a hexagon.
Substitute the given length.
Example Question #32 : Geometry
The perimeter of a regular hexagon is 
To begin, calculate the side length of the hexagon. Since it is regular, its sides are of equal length. This means that a given side is 

The little triangle at the top forms an equilateral triangle. This means that all of its sides are 


Example Question #31 : Plane Geometry

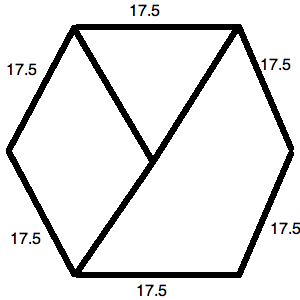
The figure above is a regular hexagon. O is the center of the figure. The line segment makes a perpendicular angle with the external side.
What is the length of the diagonal of the regular hexagon pictured above?
You could redraw your figure as follows. Notice that this kind of figure makes an equilateral triangle within the hexagon. This allows you to create a useful 

The 
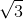


Therefore, we can say:
Solve for 
Rationalize the denominator:
Now, the diagonal of a regular hexagon is actually just double the length of this hypotenuse. (You could draw another equilateral triangle on the bottom and duplicate this same calculation set—if you wanted to spend extra time without need!) Thus, the length of the diagonal is:
Example Question #1 : How To Find The Length Of The Side Of A Hexagon
If the perimeter of a regular hexagon is 
Use the formula for perimeter to solve for the length of a side of the regular hexagon:
Where 

In this case:
Example Question #41 : Act Math
Find the length of one side for a regular hexon with a perimeter of 
Use the formula for perimeter to solve for the side length:
Example Question #42 : Act Math
Find the length of one side for a regular hexagon with a perimeter of 
Use the formula for perimeter to solve for the side length:
Example Question #43 : Act Math
What is the maximum length of each side for a regular hexago with a perimeter of 
8.8
Use the formula for perimeter to solve for the side length:
Certified Tutor
All ACT Math Resources