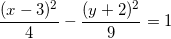Algebra II : Graphing Hyperbolic Functions
Study concepts, example questions & explanations for Algebra II
All Algebra II Resources
Example Questions
Example Question #1 : Graphing Hyperbolic Functions
In which direction does the graph of the above hyperbola open?
horizontal
vertical
horizontal
To determine which direction a hyperbola opens, first get the equation into standard form for a conic section:
This equation gives us a hyperbola when the coefficient in front of either the x-squared or the y-squared term (but not both!) is negative. In this problem, the coefficient in front of the x-squared term is positive, but the coefficient of the y-squared term is negative. Here are the rules for hyperbola directions:
- If the coefficient of the x-squared term is positive but the coefficient of the y-squared term is negative, this is a hyperbola that opens horizontally.
- If the coefficient of the x-squared term is negative but the coefficient of the y-squared term is positive, this is a hyperbola that opens vertically.
Thus, we have a graph of a horizontal hyperbola.
Example Question #2 : Graphing Hyperbolic Functions
What are the vertices of this hyperbola?
The first thing we need to find for this hyperbola is the center. This is simply the point where 
The denominator is 
Example Question #2 : Graphing Hyperbolic Functions
Write the expression for this hyperbola in standard form:
The standard form of a hyperbola is
or the similar form with a positive 
the coefficient of 
and similarly with 
and so our starting expression can be written as
Dividing by 
Example Question #1 : Graphing Hyperbolic Functions
Find the vertices of the following hyperbolic function:
We start by noticing that our hyperbola is given in the following form:
In order to determine the vertices of the hyperbola, we must first locate its center. Using the standard form given above, we know the center of the hyperbola occurs at the point (h,k), so for the equation given in the problem the center is at (2,-3). Now that we know the location of the hyperbola's center, our next step is to determine how far the vertices are from the center of the hyperbola. Looking at our equation, we can see it is in the form where the x term occurs first, which means the hyperbola opens left and right as opposed to up and down (which would be the case if the y term occurred first. Given this information, we know the vertices of the hyperbola are going to be a distance 
Certified Tutor
All Algebra II Resources
