To find the area of this pentagon, divide the interior of the pentagon into a four-sided rectangle and two right triangles. The area of the bottom rectangle can be found using the formula:

 



The area of the two right triangles can be found using the formula: 





Since there are two right triangles, the sum of both will equal the area of the entire triangular top portion of the pentagon.

Thus, the solution is:

To find the area of this pentagon, divide the interior of the pentagon into a four-sided rectangle and two right triangles. The area of the bottom rectangle can be found using the formula:

 



The area of the two right triangles can be found using the formula: 





Since there are two right triangles, the sum of both will equal the area of the entire triangular top portion of the pentagon.

Thus, the solution is:

By definition a regular pentagon must have  equal sides and  equivalent interior angles. Since we are told that this pentagon has a side length of  inches, all of the sides must have a length of  inches. Additionally, the question provides the length of the apothem of the pentagon--which is the length from the center of the pentagon to the center of a side. This information will allow us to divide the pentagon into  equivalent interior triangles. Each triangle will have a base of  and a height of . 

The area of this pentagon can be found by applying the area of a triangle formula:



 

Note: the area shown above is only the a measurement from one of the five total interior triangles. Thus, to find the total area of the pentagon multiply:

 

By definition a regular pentagon must have  equal sides and  equivalent interior angles.

This question provides the length of the apothem of the pentagon--which is the length from the center of the pentagon to the center of a side. This information will allow us to divide the pentagon into  equivalent interior triangles. Each triangle will have a base of  and a height of . 

The area of this pentagon can be found by applying the area of a triangle formula: 






Note:  is only the measurement for one of the five interior triangles. Thus, the final solution is: 

To solve this problem, first work backwards using the perimeter formula for a regular pentagon: 







Now you have enough information to find the area of this regular triangle. 
Note: a regular pentagon must have  equal sides and  equivalent interior angles. 

This question provides the length of the apothem of the pentagon—which is the length from the center of the pentagon to the center of a side. This information will allow us to divide the pentagon into  equivalent interior triangles. Each triangle will have a base of  and a height of . 

The area of this pentagon can be found by applying the area of a triangle formula: 





Thus, the area of the entire pentagon is:

By definition a regular pentagon must have  equal sides and  equivalent interior angles. 

This question provides the length of the apothem of the pentagon—which is the length from the center of the pentagon to the center of a side. This information will allow us to divide the pentagon into  equivalent interior triangles. Each triangle will have a base of  and a height of . 

The area of this pentagon can be found by applying the area of a triangle formula: 






Keep in mind that this is the area for only one of the five total interior triangles. 

The total area of the pentagon is:

To solve this problem, first work backwards using the perimeter formula for a regular pentagon: 







Now you have enough information to find the area of this regular triangle. 
Note: a regular pentagon must have  equal sides and  equivalent interior angles. 

This question provides the length of the apothem of the pentagon—which is the length from the center of the pentagon to the center of a side. This information will allow us to divide the pentagon into  equivalent interior triangles. Each triangle will have a base of  and a height of . 

The area of this pentagon can be found by applying the area of a triangle formula: 





To find the total area of the pentagon multiply:

By definition a regular pentagon must have  equal sides and  equivalent interior angles. 

This question provides the length of the apothem of the pentagon—which is the length from the center of the pentagon to the center of a side. This information will allow us to divide the pentagon into  equivalent interior triangles. Each triangle will have a base of  and a height of . 

The area of this pentagon can be found by applying the area of a triangle formula: 






However, the total area of the pentagon is equal to: 

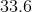
By definition a regular pentagon must have  equal sides and  equivalent interior angles. 

This question provides the length of the apothem of the pentagon—which is the length from the center of the pentagon to the center of a side. This information will allow us to divide the pentagon into  equivalent interior triangles. Each triangle will have a base of  and a height of . 

The area of this pentagon can be found by applying the area of a triangle formula: 






Note:  is only the measurement for one of the five interior triangles. Thus, the solution is: 

To solve this problem, first work backwards using the perimeter formula for a regular pentagon: 







Now you have enough information to find the area of this regular triangle. 
Note: a regular pentagon must have  equal sides and  equivalent interior angles. 

This question provides the length of the apothem of the pentagon—which is the length from the center of the pentagon to the center of a side. This information will allow us to divide the pentagon into  equivalent interior triangles. Each triangle will have a base of  and a height of . 

The area of this pentagon can be found by applying the area of a triangle formula: 





Thus, the area of the entire pentagon is: